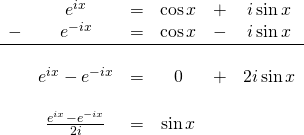Euler’s Formula

Contents

Statement of Euler’s Formula

Euler’s formula is an extremely important formula that has many uses in mathematics and all of its applications. A statement of this formula is as follows:

e^{ix}=\cos x + i\sin x

Proof of Euler’s Formula

Using Taylor series

To prove Euler’s formula, we make use of the Taylor series expansions of ex, cosx, sinx and eix

e^x \approx 1 + x + \frac{x^2}{2!} +\frac{x^3}{3!} + \frac{x^4}{4!} +\frac{x^5}{5!} +\frac{x^6}{6!} +\frac{x^7}{7!} + \dots

\cos x \approx 1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} + \dots

\sin x \approx x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \dots

e^{ix} \approx 1 + ix + \frac{(ix)^2}{2!} +\frac{(ix)^3}{3!} + \frac{(ix)^4}{4!} +\frac{(ix)^5}{5!} +\frac{(ix)^6}{6!} +\frac{(ix)^7}{7!} + \dots

Before we proceed, recall that i=\sqrt{-1}. Therefore,

  • i^2=-1
  • i^3=-i
  • i^4=-1^2=1
  • i^5=i^2\cdot i^3=(-1)(-i)=i
  • i^6=(i^3)^2=-1
  • i^7=i\cdot i^6=-i

Utilizing this information in our expression for e^{ix}:

e^{ix} \approx 1 + ix + \frac{-1x^2}{2!} +\frac{-ix^3}{3!} + \frac{1x^4}{4!} +\frac{ix^5}{5!} +\frac{-1x^6}{6!} +\frac{-ix^7}{7!} + \dots

Rearranging terms, we get

e^{ix} \approx \underbrace{1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} + \dots}_{\cos x} + i\underbrace{\left(x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \dots\right)}_{\sin x}

So

e^{ix} \approx \cos x + i\sin x

As discussed in the page on this site dealing with the Taylor’s Series, to prove that e^{ix} equals its Taylor series, we would have to prove that the error, R, between e^{ix} and its Taylor series goes to 0 as the number of terms in the Taylor series goes to infinity. Since the maximum value that the (n+1)^{\text{th}} derivative of e^{ix} can take is 1, we have

\begin{array}{rcl} \left| R \right| &\leq& \frac{M}{(n+1)!}\left| x^{n+1} \right|\\ \, &\,& \, \\ \left| R \right| &\leq& \frac{1}{(n+1)!}\left| x^{n+1} \right|\\ \end{array}

So

\lim_n\to\infty \left| R \right| &\leq& \lim_n\to\infty\frac{\left| x^{n+1}\right|}{(n+1)!} = 0

This is true for any value of x we take the Taylor series around. Therefore, e^{ix} equals its Taylor series for all x.

Using derivative of Euler’s equation

Proof taken from http://math2.org/math/oddsends/complexity/e%5Eitheta.htm

If f(x) = \cos x + i\sin x then the derivative of e^{ix} is

f^\prime(x) = -\sin x + i\cos x = if(x)

Define a function, g(x) with the property that, just like f(x)

\frac{dg}{dx} = ig(x)

Now we solve this equation.

\frac{dg}{g(x)} = i\,dx

\int \frac{dg}{g(x)} = \int i\,dx

\ln \left| g \right| = ix + C

e^{\ln \left| g \right|} = e^{ix} + C = e^Ce^{ix}

Let e^C = C_2. We get

\left| g \right| = C_2e^{ix}

g = C_3e^{ix}

We need to see if there is any value of the constant, C_3, that makes f(x) = g(x). Set x=0. We have

f(0) = \cos 0 + i\sin 0 = 1 + 0 = 1

g(0) = C_3e^{i0} = C_3e^0=C_3

So g(x) = f(x) when C_3 = 1. Plug 1 into the equation for g(x)

g(x) = 1\cdot e^{ix} = e^{ix} = f(x)

Corollaries

There are 2 important corollaries that can be derived directly from Euler’s formula. To prove these corollaries, we need 2 trigonometric identities:

  • \cos -x = \cos x
  • \sin -x = -\sin x

A diagram that illustrates these identities can be found at the following site:

https://www.themathpage.com/aTrig/functions-angle.htm#theorem2

Cosine in terms of exponentials

Euler’s formula states:

e^{ix} = \cos x + i\sin x

Now substitute -ix for ix. We get

e^{-ix} = \cos -x + i\sin -x = \cos x - i\sin x

Now add the 2 equations:

\begin{array}{cccccc} \, & e^{ix} & = & \cos x & + & i\sinx\\ + & e^{-ix} & = & \cos x & - & i\sinx\\ \hline\\ \, & e^{ix} + e^{-ix} &=& 2\cos x &+& 0\\ \, &\,& \,& \, & \, & \, \\ \, & \frac{e^{ix} + e^{-ix}}{2} & = & \cos x &\,& \, \end{array}

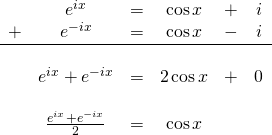
Sine in terms of exponentials

To prove this corollary, we perform subtraction on the 2 equations we added above:

\begin{array}{cccccc} \, & e^{ix} & = & \cos x & + & i\sin x\\ - & e^{-ix} & = & \cos x & - & i\sin x\\ \hline\\ \, & e^{ix} - e^{-ix} &=& 0 &+& 2i\sin x\\ \, &\,& \,& \, & \, & \, \\ \, & \frac{e^{ix} - e^{-ix}}{2i} & = & \sin x &\,& \, \end{array}